readme.md

572. Subtree of Another Tree

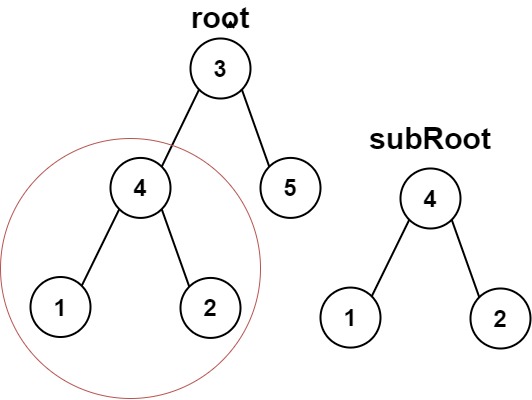
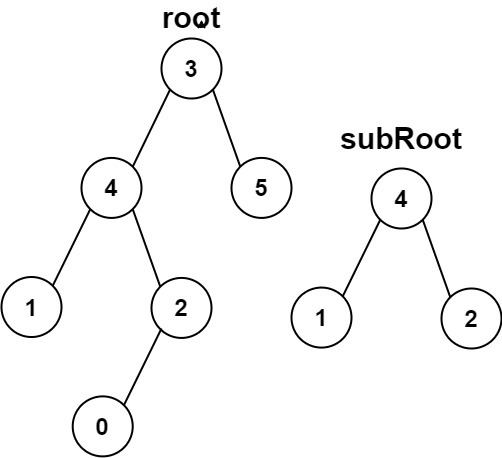
Given the roots of two binary trees root and subRoot, return true if there is a subtree of root with the same structure and node values of subRoot and false otherwise.

A subtree of a binary tree tree is a tree that consists of a node in tree and all of this node's descendants. The tree tree could also be considered as a subtree of itself.

Example 1:

Input: root = [3,4,5,1,2], subRoot = [4,1,2] Output: true

Example 2:

Input: root = [3,4,5,1,2,null,null,null,null,0], subRoot = [4,1,2] Output: false

Constraints:

• The number of nodes in the root tree is in the range [1, 2000].
• The number of nodes in the subRoot tree is in the range [1, 1000].
• -104 <= root.val <= 104
• -104 <= subRoot.val <= 104

Constraints:

• The number of nodes in both trees is in the range [0, 100].
• -104 <= Node.val <= 104

Solution

class TreeNode:
    def __init__(self, val=0, left=None, right=None):
        self.val = val
        self.left = left
        self.right = right

class Solution:
    def isSubtree(self, root: Optional[TreeNode], subRoot: Optional[TreeNode]) -> bool:
        if not root:
            return False

        # Check if the current subtree matches subRoot
        if self.isSameTree(root, subRoot):
            return True

        # Recursively check the left and right subtrees
        return self.isSubtree(root.left, subRoot) or self.isSubtree(root.right, subRoot)

    def isSameTree(self, p: Optional[TreeNode], q: Optional[TreeNode]) -> bool:
        if not p and not q:
            return True
        if not p or not q:
            return False
        if p.val != q.val:
            return False
        return self.isSameTree(p.left, q.left) and self.isSameTree(p.right, q.right)

Thoughts

Time Complexity

The time complexity is O(m * n), where m is the number of nodes in the root tree and n is the number of nodes in the subRoot tree. In the worst case, we might have to compare subRoot with every subtree of root.

Space Complexity

The space complexity is O(h), where h is the height of the root tree. This is due to the recursive call stack. In the worst case, the tree could be linear (i.e., a linked list), in which case the height of the tree would be equal to the number of nodes, leading to a space complexity of O(m).